Scientific Notes on Power Electronics: PNP and NPN Transistors

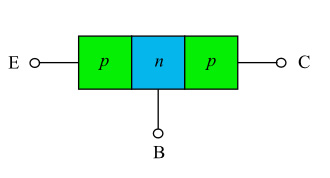
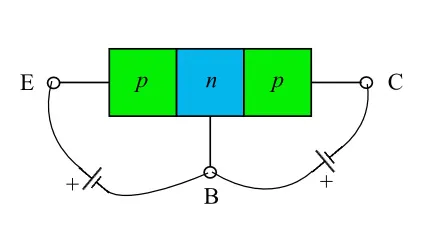
If we try to solder a pn junction to an np junction, we obtain the device shown in Figure 1, where the letters  E, B, and C mean Emitter, Base, and Collector, respectively. These are the names assigned to the three regions p, n, p.

We have thus created a PNP transistor.

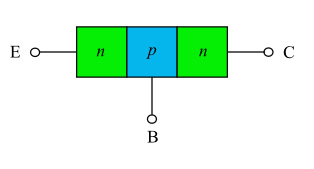
If instead, we solder an np junction to a pn junction, we have the device shown in Figure 2, in which the roles of E, B, and C, concerning doping, are reversed. The result is an NPN transistor. In both configurations, the device consists of junctions J_E and J_C.

The transistor is also known as a semiconductor triode because it marked the end of the vacuum tube, just as the junction diode replaced the vacuum diode. Another common name is BJT, an acronym for Bipolar Junction Transistor¹.  The bipolar attribute refers to the electrical conductivity in semiconductors (given by electrons and holes).

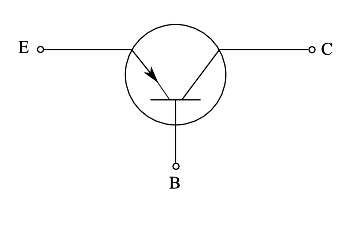
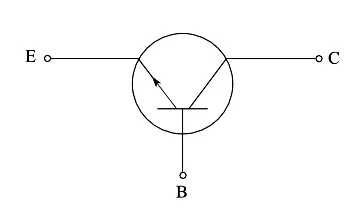
In Figure 3 we can see the circuit symbol of the PNP transistor, presented in such a way as to highlight the relationship with the diagram in Figure 1. Similarly, Figure 4 shows the NPN transistor symbol.

Open-circuit transistor

Let’s refer to a PNP transistor (the results immediately generalize to an NPN transistor). In open circuit conditions, we expect behavior similar to that of a single junction, i.e. the presence of a contact potential (see a previous tutorial) which is actually a potential energy barrier whose purpose is that of preventing the diffusion of holes from the emitter to the base. If there were no such barrier, the holes would spread indefinitely towards the base; manifestly non-physical situation as we are in open circuit conditions. If V₀ is the height of the barrier at the junction J_E, similar arguments will lead us to say that a barrier V′ exists on the junction J_C. Assuming the individual regions p,n,p identical with the same concentration of impurities, we have V₀ = V′.

The achievement of thermal equilibrium will be characterized by constant values of the concentrations of the minority carriers of the single region:

Having used the following convention: the main letter denotes the carrier (electron, hole), and the first subscript indicates the region it belongs to. For example, the symbol n_p tells us that we are considering electrons in a p region (hence minority charges). Finally, the third subscript 0 indicates that the system is in thermodynamic equilibrium at temperature T₀ (which is not necessarily room temperature).

It should be noted that we are neglecting the depletion layer (discussed in a previous tutorial), as it is of negligible size compared to the double junction layer.

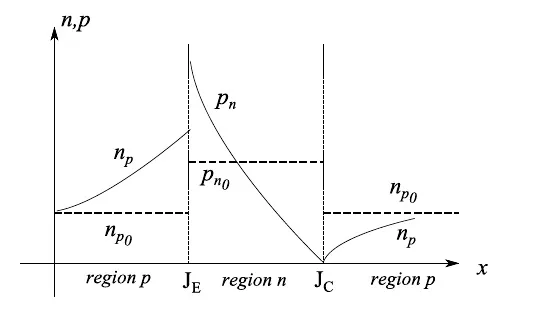
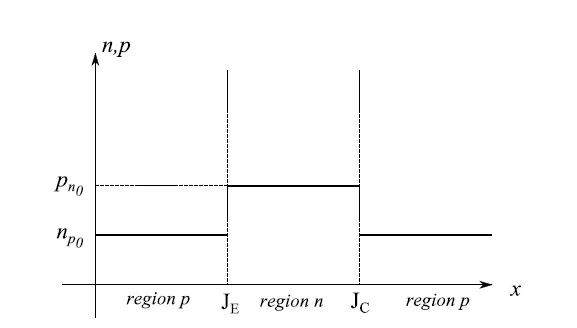
In the p-type region, the concentration n is the same because we have assumed these regions to be identical. If in a system of Cartesian axes, we report on the abscissa the linear dimensions of the double junction that constitutes a PNP transistor, and on the ordinate the concentrations of minority carriers in the single region (equation (1)), at the thermodynamic and open circuit equilibrium we will have a trend like that shown in Figure 5, where we assumed p_n0 > n_p0.

It should also be noted that the trends, strictly constant, in the concentrations of minority carriers and in open circuit conditions, are the result of an averaging operation since these quantities fluctuate around an average value, which is the measured one.

Transistor bias

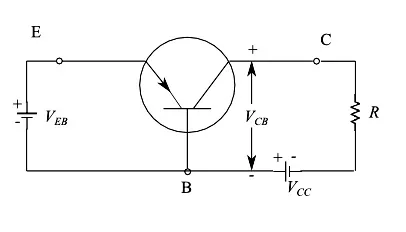
There are more polarization combinations than the diode, thanks to the presence of two junctions and three terminals.  As usual, let’s refer to the PNP transistor, directly biasing J_E and J_C inversely. In Figure 6 we report this polarization configuration, while Figure 7 is the corresponding circuit diagram. Precisely, we have a voltage generator with V_EB, while V_CC indicates the voltage generated by the battery connected to J_C. With V_CB we instead indicate the potential difference between collector and base.

Forward biasing of J_E reduces the potential barrier (as established in the previous section); this causes diffusion of holes from the emitter to the base and of electrons from the base to the emitter. The result is, therefore, a macroscopic increase in the concentrations of minority carriers in the two regions p and n of J_E.

Let’s focus our attention on the widespread holes in the base. Here, we are near J_C with the following electric charge configuration: if we measure the potential difference between C and B, we find a value as indicated in Figure 7, and this means that the holes are “collected” by the collector C. However, the J_C is inversely biased, and this determines an exponential damping of the holes in the base so that at J_C the concentration of these carriers is statistically zero. In turn, the reverse bias of J_C causes a reduction in the electron concentration in the p-region of J_C, which will dampen exponentially on J_C. As stated previously, the concentration of electrons in the p-region of J_E undergoes an increase which will then dampen exponentially due to the well-known diffusion mechanism of minority carriers in a junction. These conclusions are summarized in Figure 8.